How to Turn Four Notes Into Nearly Infinite Musical Possibilities

Permutation – “often major or fundamental change (as in character or condition) based primarily on rearrangement of existent elements.”

The new JodyJazz DV HR Tenor uses the patented DV secondary window to add mid and low harmonics, resulting in a more authoritative tone than normal tenor sax mouthpieces provide. And the long straight somewhat high baffle gives the mouthpiece it’s fantastic projection and cutting power along with excellent altissimo.

JODYJAZZ.COM

The New DV HR Tenor from JodyJazz

“the act or process of changing the lineal order of an ordered set of objects.” – Merriam-Webster

Thank you Merriam. You hit the nail on the head.

The “rearrangement of existent elements” and the “ordered set of objects” in question here in our case is none other than the four-note grouping (tetrachord) formed by steps 1, 2, 3 & 5 of the Major Scale (C–D–E–G in C Maj.).

Whether played or sung, and regardless of style, the 1235 Major tetrachord is probably the most commonly heard, used and recognized group of 4 notes in Western music. It’s closely related to the Major Pentatonic scale (C–D–E–G-A – one note short), which is itself based on the Cycle of Fifths (C-G-D-A-E) – and therefore the natural series of overtones.

“So,” you ask, “why the fascination with 4 notes instead of 5?”

Well, from a purely mathematical standpoint, any group of four separate, individual items (notes in this case) yields 24 unique arrangements of those four items, while with a group of 5, that number increases five-fold, to 120.

Now, I don’t know about you – but even 24 possibilities seems almost unmanageable, let alone 120!

Another reason is that since most Western music, especially Jazz and Pop, is in some type of 4/4 time, four-note groupings of eighth and / or sixteenth notes became the standard division – especially, for example, in post WWII Jazz. These groupings became known as “digital patterns” (that’s digital as in “finger”). The great John Coltrane, among others, was a purveyor of this device at certain points in his career.

Now that we’ve gotten that out of the way, the below graphics tell us what the 24 permutations of 1235 and and their corresponding letter names – CDEG in C Major – look like.

Ex.1 – The 24 Permutations of 1235

(1,2,3,5) (1,2,5,3) (1,3,2,5) (1,3,5,2) (1,5,2,3) (1,5,3,2)
(2,1,3,5) (2,1,5,3) (2,3,1,5) (2,3,5,1) (2,5,1,3) (2,5,3,1)
(3,1,2,5) (3,1,5,2) (3,2,1,5) {3,2,5,1) (3,5,1,2) {3,5,2,1)
(5,1,2,3) (5,1,3,2) (5,2,1,3) (5,2,3,1) (5,3,1,2) (5,3,2,1)

Ex.2 – The 24 Permutations of CDEG (Scale Steps 1235 in C Major)

(C,D,E,G) (C,D,G,E) (C,E,D,G) (C,E,G,D) (C,G,D,E) (C,G,E,D)
(D,C,E,G) (D,C,G,E) (D,E,C,G) (D,E,G,C) (D,G,C,E) (D,G,E,C)
(E,C,D,G) (E,C,G,D) (E,D,C,G) (E,D,G,C) (E,G,C,D) (E,G,D,C)
(G,C,D,E) (G,C,E,D) (G,D,C,E) (G,D,E,C) (G,E,C,D) (G,E,D,C)

“Permutation Station 1-2-3-5” presents all the above permutations in notation form, as well (of course).

The 1235 tetrachord, in root position, contains the following interval make-up:

• two Major 2nds (C-D & D-E),
• one minor 3rd (E-G),
• one Major 3rd (C-E),
• one Perfect 4th (D-G) and
• one Perfect 5th (C-G).

The sequences are organized by chapter in ascending or descending fashion by:

• half steps (Semitone Cycle),
• whole steps (Whole Tone Cycle),
• minor 3rds (Diminished Cycle),
• Major 3rds (Augmented Cycle), and
• the Cycle of Fourths (5ths)

Playing them through these cycles,.not only facilitates technique and ear training, it’s also much more interesting than if you would simply play them straight up, staying within one key center, which would be monotonous – to say the least.

It will also familiarize you with the different possible melodic & harmonic connections and resolution points between them, as happens with an actual tune.

Ex.3 – Minor 3rds Cycle – Permutation 2153a & 3521a

Ex. 3 above shows a 2153 permutation ascending in minor 3rds (Diminished Cycle), as well as its descending retrograde 3512 The Diminished Cycle splits the octave into to 4 equal parts. Hence, the a, b, c & d designations.

Since any note of a 1235 can be altered (e.g. 1 b2 3 5, 1 2 b3 b5, etc.), as in any other scale or chord, in order to fit a particular harmony, altered or not, I became curious to see if it might be possible to employ unaltered 1235 groupings in order to create altered tensions within chords with different roots. For example, 1235 in Eb over G = G7#9 b13, or 1235 in Db over G = G7b5 b9 b13 – or both – which would contain all 4 possible altered tensions (G7alt), as in bar #2 in Ex. 4 below.

Ex.4 – Multiple unaltered 1235 Permutations (w/ inversions) as altered extensions in a ii-V7-I melodic line.

Which leads us to our next stop: “Permutation Station II – Next Station: Inversions“, which in turn takes a closer look at the 1st, 2^nd and 3rd inversions of each individual permutation. Along with the original root positions already examined, a total of 96 (4 x 24) unique melodic possibilities per key are up for examination.

Possibly, for the first time anywhere, this book explores the possibilities, as in Ex. 4, of using both single and multiple unaltered 1235 units as melodic material to fit any chord type; a handy tool for improvisers and composers alike

The graphic below depicts three different 1235 permutations of CDEG, in root position, 1st, 2nd and 3rd inversions (moving from left to right).
In each case:

Root Position – C or 1 is the lowest note
1st Inversion – D or 2 is the lowest note
2nd Inversion – E or 3 is the lowest note
3rd Inversion – G or 5 is the lowest note

Although the note names change with the key, the number designations relative to their inversion remain the same.

Ex. 5 – 1235 and 1253 inversions in C

Ex. 6 illustrates how a common tetrachord (the same four notes – CDEG) with varied permutations and inversions, might be employed effectively over a changing harmonic landscape.

The numbers underneath each staff represent its permutation. Those in parenthesis represent the inversion.

Ex. 6 – “A Jiggy Little Ditty” (B. Stern)

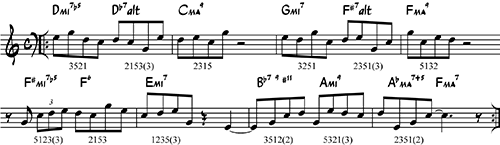
Listen to ex. 6

As in Ex. 4, the ii-V7-I line in C in Ex. 7 below employs multiple unaltered 1235 permutations.

In bar #1, the two root position permutations from F & Ab respectively, combine to melodicise the Dmin7b5 ii chord.

In bar #2 = G (3^rd inversion) & Eb = G7#9 13,

bar #3 = C (1^st inversion) & G (2^nd inversion) = C Maj7

bar #4 = Bb & Ab (both 1^st inversion). It might be helpful to think of each bar’s groupings in pairs.

Ex. 7 – ii-V7-I line employing multiple unaltered 1235 Permutations (w/ inversions) as altered extensions.

Listen to ex. 7

It’s interesting to note the different intervallic relationship between groupings in each measure above – min 3^rd, Maj 3^rd, Perfect 5^th & Maj 2^nd, respectively. These are all cycle sequences which are covered in the initial volume, “Permutation Station 1-2-3-5” .

Ex. 8 – One more ii-V7-I for the road.

Listen to ex 8.

See if you can determine the “key centers” in Ex. 8 above.

The ii-V7 sequences found in either volume were not conceived in a preordained, formulaic manner. Rather, they were built using different combinations of permutations, inversions and interval spacing between their respective “key centers”.

The only two basic rules, which I tried not to breach for each 4-note grouping were:

1. Limit the range in each case, from bottom note to top, to an octave, and
2. Hold the integrity of each four-note group of eighth-notes intact – meaning foreign notes or rests in between are a no-no – all four notes must be present and form an uninterrupted unit. Triplets are fine as long as the previous conditions are met. Exceptions can be made in the case of static chords which last several beats or more.

This all might seem to some of you like trying to fit a square peg into a round hole…

But hey – it works!

CLICK HERE TO DOWNLOAD THE SHEET MUSIC EXAMPLES IN A MORE READABLE AND PRINTABLE PDF FORMAT.